Hemispherical Reflectance and Emittance Properties of Carbon Nanotubes Coatings at Infrared Wavelengths

Description: In this NASA – Goddard paper the hemispherical reflectance and emmitance properties at IR wavelengths of experimental nano-tube coatings are compared with Acktar Fractal Black. A Fractal Black coated disc is used as a “black standard” in the measurements
Hemispherical Reflectance and Emittance Properties of Carbon Nanotubes
Manuel A. Quijada, John G. Hagopian, Stephanie Getty, Raymond (Robin) E. Kinzer, Jr., and
Edward J. Wollack
Goddard Space Flight Center, 8800 Greenbelt Rd., Greenbelt, MD 20771;
ABSTRACT
Recent visible wavelength observations of Multiwalled Carbon Nanotubes (MWCNT) coatings have revealed that they represent the blackest materials known in nature with a Total Hemispherical Reflectance (THR) of less than 0.25%.
This makes them exceptionally good as absorbers, with the potential to provide order-of- magnitude improvement in stray-light suppression over current black surface treatments when used in an optical system.
Here we extend the characterization of this class of materials into the infrared spectral region to further evaluate their potential for use on instrument baffles for stray-light suppression and to manage spacecraft thermal properties through radiant heat transfer process.
These characterizations will include the wavelength-dependent Total Hemispherical Reflectance (THR) properties in the mid- and far-infrared spectral regions (2-110 μm). Determination of the temperature-dependent emittance will be investigated in the temperature range of 40 to
300 K. These results will be compared with other more conventional black coatings such as Acktar Fractal Black or Z306 coatings among others.
Keywords: Multi-Walled Carbon Nanotubes (MWCNT), Hemispherical Reflectance, Emittance, Stray-light.
INTRODUCTION
In this paper, we investigate the infrared properties of Multiwalled Carbon Nanotube (MWCNT) coatings. This is a follow up study to our earlier effort primarily aimed at developing these nanostructure materials for stray light and diffraction suppression at visible wavelengths.
1 Our group has found that MWCNT are exceptionally good absorbers, with potential to provide order-of-magnitude improvements over current surface treatments and a resulting factor of 10,000 reduction in stray light when applied to an entire optical train.
Our research has been motivated by the stringent stray light control needs which occur in the realization of precision optical systems for remote sensing. Examples include earth observations by satellite imaging systems where high brightness contrast exists between desired field of view and other background sources which can illuminate and scatter from elements of the instrument.
This bright light diffracts from instrument structures, rattles around and invariably contaminates measurements. Astrophysical observations are also impacted by stray light that obscures very dim objects and degrades signal to noise in spectroscopic measurements. Stray light is controlled by utilizing low reflectance structural surface treatments and by using baffles and stops to limit this background noise.
Development of this technology will provide numerous benefits including the increase in observational fidelity by reducing scattered light in high contrast regions.
Our ob jective has been to develop and apply MWCNT to instrument components to realize the benefits mentioned above. We have addressed the technical challenges to advance the technology by tuning the MWCNT growth geometry using a variety of methods and on various types of substrates to provide a factor of 10 improve- ment in absorption at UV/visible wavelengths over current surface treatments used in space flight hardware.
 In this paper, we are exploring the potential of MWCNT as thermal-control coatings at mid- and far-infrared wavelengths by reporting characterization of the Total Hemispherical Reflectance (THR) in the 0.2 to 110 μm wavelength range. These measurements will be extended to different types of MWCNT samples grown on differ- ent substrates. These results will be used to derive the wavelength-dependent emittance properties as a function
In this paper, we are exploring the potential of MWCNT as thermal-control coatings at mid- and far-infrared wavelengths by reporting characterization of the Total Hemispherical Reflectance (THR) in the 0.2 to 110 μm wavelength range. These measurements will be extended to different types of MWCNT samples grown on differ- ent substrates. These results will be used to derive the wavelength-dependent emittance properties as a function
of temperature. We have used this type of characterization to optimize the growth process and geometry for robustness and maximum absorption. In addition to the applications mentioned above, optimization of the MWCNT samples will be pursued in detector applications to enhance light absorption in order to realize gains in detector quantum efficiency.
The paper is organized as follows: we will first present a discussion of the reflectance properties and a description of the method used to derive the temperature-dependent emittance property using an optical method. This will be followed by a description of the equipment used to measure the Total Hemispherical Reflectance (THR) quantity in all of our samples. We will then proceed to show the results of THR and emittance calculations for samples grown on various substrate. This will be followed with concluding remarks.
EXPERIMENTAL TECHNIQUE
2.1 Bi-directional Reflectance
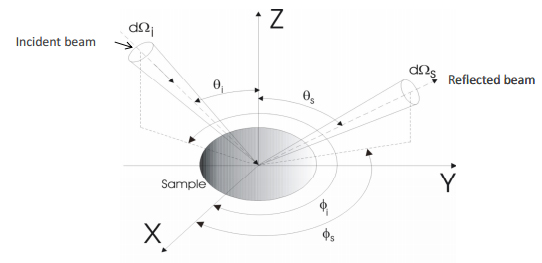
The term bi-directional reflectance ρ (θi , φi ; θs , φs ), is defined as the amount of light from a given direction θi , φi that is reflected into a specified solid angle dΩs and out going direction θs , φs as shown in the diagram of Fig. 1.
In addition, the directional reflectance ρd (θi , φi ) is defined as the ratio of the total energy reflected into the subtending hemisphere to the energy incident on the surface from the direction θi , φi . Hence, and following the notation of Nicodemus et.al.,2 the relation between directional reflectance ρd (θi , φi ) and ρ (θi , φi ; θs , φs ) is given by the integral of the latter over the viewing hemisphere:
![]()
For a perfectly diffuse isotropic reflector ρ (θi , φi ; θs , φs ) = constant, and integration of Eq. 1 gives
![]()
2.2 Emittance
The measured directional reflectance of a sample may be used to compute one important property required for radiative heat transfer analysis, e.i., the directional emittance εd. From conservation of energy principles, the directional emittance of an opaque surface at a given wavelength and angle of incidence may be obtained from
![]()
where ρd (θi , φi , λ) is the measured directional reflectance as a function wavelength λ. Equation 3 is also known as Kirchhoff ’s law and it is applied in a steady-state condition for a surface or body in thermal equilibrium. For the remaining discussion we will omit the θi and φi dependence of ρd and εd for brevity. From the relation in Eq. 3 and performing an integral over λ, the total directional emittance (εt ) of the surface at a given temperature may be found from:
![]()
where
![]()
is the Planck’s distribution function for the given wavelength (λ) and temperature (T ). Substituting values for the Planck’s constant h, speed of light c, Boltzman constant k, and providing the appropriate unit conversion so λ can be expressed in μm, we find
![]()
The equations above allow emittance determination for various instances depending on the angular coverage of the reflectance measurements. The first instance is directional angular emittance when reflectance is measured at any angle of incidence including near-normal (θ ?? 10◦) reflectance. The second case is spectral hemispherical emittance εH (λ), when reflectance has been measured over a sufficiently wide range of incident angles to permit integration over the entire hemisphere,
![]()
Finally, total hemispherical emittance εH , when reflectance has been measured over a sufficiently wide range of incident angles to permit integration over the hemisphere,
![]()
Finally, it should also be noted that for diffuse and isotropic reflectors εt (θ) = constant and, in this limit, the integral in Eq. 8 yields εH = εt . This is what would be expected for a Lambertian surface.
2.3 Total Hemispherical Reflectance
For specular reflectance, the angle of reflection is equal to the angle of incidence on the substrate; direct reflection off the surface is carefully controlled. In most optical instruments the source of stray light that is more difficult to control is the diffusely scattered light at all angles.
This light can be reflected many times and find its way to the instrument focal plane where it can degrade the observations by creating noise that obscures the signal of interest. Characterization of this scattered light leads us to the concept of Total Hemispherical Reflectance (THR) which is the reflectance measured of all light that is scattered off of a test sample at all angles.
The measurement of THR does not discriminate the angle of reflectance; only the total amount of reflected light. It is also a good measure of the relative ability of materials to absorb light and therefore it could be an effective screening tool to determine the effectiveness of a particular MWCNT growth process. However, it does not provide the full range of characterization required for modeling an instrument to determine system stray light.
Our chosen method for measuring THR is with an integrating sphere where an incoming beam illuminates a test sample attached onto the sphere from a given direction and the radiation scattered by the sample is collected and detected.
The results reported here on the MWCNT samples were obtained over a spectral range from 0.2 to 110 μm. Collection of data over such a wide range required the use of up to 3 instruments. The UV/Vis/NIR spectral range from 0.2 to 2.5 μm was measured using a Perkin Elmer Lambda 950 equipped with a 60 mm diameter integrating sphere.
The light detection is done by two detectors located inside the sphere: A lead sulfide (PbS) detector to cover the 0.860-2.500 μm region and a R955 photomultiplier tube (PMT) for
the wavelength range between 0.200 and 0.860 μm. Finally the inside of the sphere is coated with a Spectralon coating.
For coverage at longer wavelengths, we used a Bruker Fourier Transform Infrared (FTS) spectrometer with an integrating sphere accessory that is fitted with a room-temperature DTGS detector to provide coverage in the 2 to 20 μm wavelength range.
A second integrating sphere was used to obtain THR data from 20 to 110 μm. This accessory is equipped with a 4.2 K detector bolometer and it is capable of providing THR data at cryogenic temperatures (5 to 100 K). Operational features and construction details for these two integrating sphere accessories will be provide in the following sections.
2.4 Bruker FTS Integrating Sphere Reflectometer
The Bruker FTS (IFS125HR) was the instrument of choice to collect data in the 2 to 20 μm spectral range at ambient temperature (300 K). Adequate experimental conditions for measurements in this spectral range were achieved by choosing combinations of the following components: tungsten lamp or Globar source, Si/CaF2 or Ge/KBr beam splitter and a DTGS detector with a KBr window.
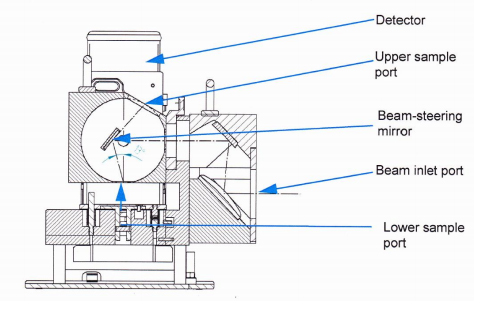
The reflectance attachment used for these measurements was mounted in the sample compartment of the spectrometer and it consists of a hollow sphere (75 mm diameter) coated with an electro-deposited Lambertian gold coating on the inside. As the Fig. 2 shows, the sphere has one beam inlet port, two sample ports and one outlet port allowing the light to reach the detector after the integration.
The infrared (IR) beam enters the integrating sphere through the beam inlet port and impinges on the beam-steering mirror which is mounted inside the sphere slightly off center. Depending on the position of the beam-steering mirror, the IR-beam is directed either to the upper or lower sample port.
In either case, the angle of incidence for the IR-beam impinging on the sample (or reference) is 13◦. In this configuration, the specular and the diffusely reflected radiation hits the sphere wall at least once and, therefore, both are included in the flux measurements. In this arrangement no baffles are needed.
2.5 OPASI-T Cryo-Reflectometer
For measurements in the far-infrared spectral region (20 to 110 μm) we used a reflectometer fitted to a second Bruker FTS spectrometer (IFS113). Figure 3 shows a diagram of this reflectometer that is uniquely suited for performing hemispherical reflectance measurements at cryogenic temperatures (5 to 100 K).
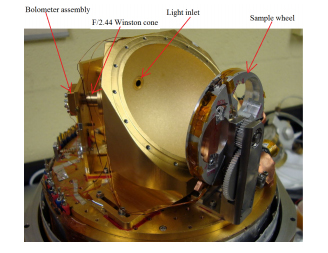
The integrating sphere itself is machined aluminum with the interior surface being bead blasted with 16-grit SiC, creating an
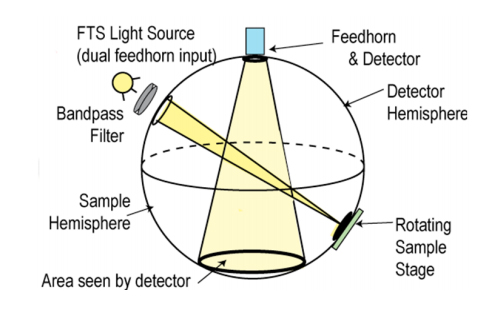
approximately isotropic scattering surface, and gold-plated to maximize surface reflectivity (see Fig. 4). Light enters the sphere through a double-ended f/6 Winston cone; this provides illumination to a small patch (21.8 mm diameter) on the target. The input light is either a mercury arc or a glow-bar that comes from inside the Bruker FTS.
Three targets sit within a three-position sample wheel, allowing us to measure in sequence a black (absorptive) target, a reflective target, and the test sample. The wheel also has a magnetic switch that allows accurate tracking of the rotation position.
Light reflected off this test sample then scatters inside the sphere to an f/2.44 Winston cone that brings the light to an Infrared Laboratory Inc. (IR-Lab) cooled Si bolometer. This cone is positioned so that light from the sample cannot directly reflect from the sample into the detector Winston cone; this eliminates the need for baffling inside the sphere. The sphere and bolometer are mounted to the cold plate of a liquid Helium cryostat.
The sample wheel is thermally isolated from the cold plate with a stainless steel support bracket, and has a mechanical heat switch to provide the ability to thermalize the wheel.
Mounted to the wheel are both a thermometer and a 300 Ω resistive heater; the heater allows the wheel, when isolated, to be temperature controlled between ~4.2 K and ~100 K. Thermal radiation from the wheel provides the limit at the upper end of this scale, as when the wheel temperature exceeds 100 K, it radiatively heats the bolometer.
3. MWCNT GROWTH AND OPTIMIZATION
The growth of high-absorption low hemispherical reflectance MWCNT samples has been achieved through three phases. The first phase consisted of tuning the nanotube geometry to produce the desired low reflectance over all angles on Si.
Next, we worked on improving adherence of the MWCNT layers onto Si. Finally, we have worked on nanotube deposition on alternate substrate materials in order to broaden the scope of application, such as improved components for stray light suppression and for use as infrared detector absorbers.
3.1 MWCNT Growth on Si
Fabrication of vertically oriented MWCNT films was accomplished by catalyst-assisted chemical vapor deposition (CVD).
Using Si as the growth substrate, the fabrication begins with the thermal deposition of aluminum/iron thin film catalyst. To grow MWCNT, the substrate is exposed to ethylene feedstock gas at 750◦C in a reducing environment; the ethylene is dissociated at the iron surface, and the carbon is extruded in the form of a dense film of aligned MWCNT. Precise patterning of the MWCNT film can be accomplished by constraining the placement of the catalyst film through conventional lithographic means.
Varying the catalyst thickness on the substrate can modulate the MWCNT height. We also used the modulation of catalyst film thickness to produce low-density,
tall MWCNT. We studied the extreme regime of catalyst thickness to characterize the limits of this technique to producing low MWCNT fill factors.
Minimization of catalyst film thickness resulted in an order of magnitude improvement in the performance of the MWCNT as an absorber. Our process resulted in MWCNT with inner diameters of 1-5 nm, outer diameters of 30-100 nm and average spacing of 100-500 nm.
The best samples had lengths of 50 to 100 μm, but it is highly probable that longer lengths would provide better performance.
4 We have also recognized the importance of carbon nanotube adhesion given the environmental conditions associated with end usage. Other properties we have considered are how straight the fibers lie, their heat capacity, charging properties in a radiation environment, and others. The adhesion question is discussed in more detail in the following section.
3.2 Adhesion Enhancement on Si
Carbon nanotubes grown on Si with only the iron catalyst layer exhibit poor adhesion; it is quite easy to rub the nanotubes off of the substrate with any contact. This poor adhesion severely limits the utilization of MWCNT in space flight hardware.
Previous work suggests that the point of failure is at the catalyst-substrate interface. We explored alternative substrate preparation techniques to improve adhesion.
The primary approach we developed was to use a thin-film adhesion layer under the iron catalyst layer. We experimented with chromium, titanium and alumina underlayers. The approach was straightforward and should not have significantly impacted the favorable properties of the thin Fe catalyst film.
What we found however was that the nanotube growth properties were significantly affected by this layer. It was determined that alumina provided a good adhesion layer without degrading the optical properties of the carbon nanotubes.
3.3 Growth on alternate materials
As mentioned above, the nanotubes films are typically grown on Si substrate. Although many optical components including mirrors, slits and small blocking elements such as coronographic masks could be feasibly made out of Si, the use of this substrate alone will limit the usability of the nanotube coatings in situations where it is required to use more robust substrates.
We investigated and developed a growth process that yielded similar performance as samples grown on Si. The substrates investigated included stainless steel, nickel and titanium using the same thickness of iron catalyst layer and growth parameters optimized on the Si substrates. Titanium substrates produced low THR when the adhesion layer thickness was increased.
Successful growth using the optimized process was also achieved on stainless steel. We are also exploring nanotube growth on silicon nitride (SiN) membranes for infrared detector absorber structure applications.
The spectral neutrality over an extremely large bandwidth is where the promise of a carbon nanotube absorber approach could be of value in the mid-
RESULTS
4.1 THR Data at Ambient Temperature
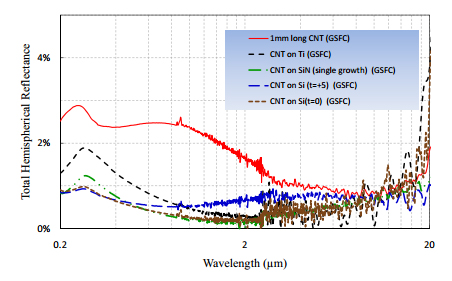
Figure 5 shows THR data for several nanotube samples grown on different substrates. The sample with the highest THR values in the short wavelength range (below 2 μm) is the one labeled “1mm long”.
This is a sample that was grown at Southern Illinois University (by Saikat Talapatra) that was initially determined to have relatively high THR in the UV-NIR. However, measurements in the mid-infrared show that the MWCNT on this sample are a much better absorber in this region.
The silicon, SiN, and Ti substrate samples all have nanotube lengths of 50 to 100 μm. Hence, the results in Fig. 5 show that they all perform better in the UV to near-infrared but are similar to the “1mm long” sample at longer wavelengths. This is probably due to the difference in the effective index of refraction of the samples as a function of wavelength.
The initial interaction of light with the nanotube mat results in reflection based on the “effective” index change between air (or vacuum) and the nanotubes. Since carbon has a relatively high index, we want a very low density of nanotubes so that the boundary does not produce a large reflection. Bulk carbon is black but very shiny due to its high index.
The nanotube mat has about a 1% fill factor making the effective index approach 1 to prevent a high impedance mismatch. The density of the “1mm long” sample is higher, therefore it reflects more light at short wavelengths.
At longer wavelengths, the index changes similarly for all samples, but the penetration of the light into the nanotube mat begins to dominate, with a higher efficiency of absorption for the taller mats.
Therefore, best performance requires an optimization of density and height to provide best broad band absorption. Finally, we show two samples grown on Si with the growth parameter “t” being the time of hydrogen pre-treat before growth set at “t=0” and “t=+5” where the numbers are in units of minutes. The “t=0” sample grown on Si has the lowest average THR of all the samples in the spectral range shown in Fig. 5.
4.2 Far-Infrared Cryo-Reflectance
We now turn our attention to THR data obtained with the OPASI-T reflectometer on three selected samples from the set shown in Fig 5.
Before we do this, let us first discuss the procedure by which these data are obtained. As mentioned earlier, the filter wheel in the reflectometer has three positions. The first position corresponds to the highly reflecting sample that is typically made out of a polished Al disk. Measurements taken with this
sample provide the “100% baseline” calibration that will be needed later for correcting and normalizing the test sample response. A second position on the wheel is occupied by a “black” calibrator and it is used to calibrate out the “0% baseline” response. The sample under study is placed on the third and final position on the wheel.
Because of the limited nanotube sample size, we placed an aperture (made out of reflecting Al tape) to mask the oversized beam hitting the test port on the sphere. Since this mask was common to all three filter wheel positions, the oversized beam provided a relatively high background signal reading that needed to be removed from the sample results. The procedure below discusses how this normalization was carried out.
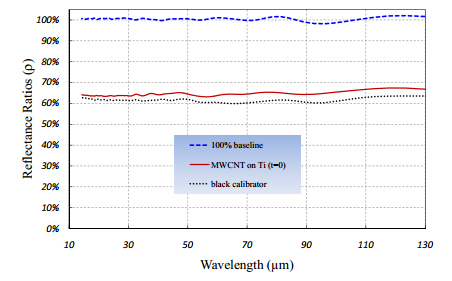
The experiment was started by first performing a cool-down of the reflectometer dewar with liquid Helium (LHe) in order to cool the bolometer detector to its operating temperature at 4.2 K. The temperature for the whole system was monitored until it reached thermal equilibrium. This was done by checking and collecting a series of scans of the FTS interferometer signal on the reflecting or “100% baseline” sample mentioned above.
The system was deemed stable after several consecutive ratios of this signal yield a nearly 100% constant line as shown by the curve labeled “100% baseline” in Fig. 6. Once the system had reached thermal equilibrium, a series of 100% reference scans were collected and averaged. After this, the filter wheel was rotated to the position of the black calibrator, an image of which is shown in Fig. 7.
This sample has a regularly textured surface that had been specifically engineered to create an absorbing or low-reflecting surface at the longest wavelength possible in the far-infrared spectral region.5 The surface is coated with a highly absorbing layer of Acktar Fractal Black to provide the maximum absorption possible at the shorter wavelengths in the far- and mid-infrared spectral regions. Independent calibrations for this sample have provide a flat hemispherical reflectance in the 2 to 110 μm range with an average value close to 1-2%.6
The ratio of this black calibrator response to that of the highly reflecting sample is shown in Fig. 6 with the curve labeled “black calibrator”. We observe this ratio has an average value close to 62%. The reason for this higher than expected “0% baseline” value is due to the fact that the sample is being illuminated by a roughly
30% oversized beam; most of this signal results from light reflections off the surrounding Al mask with a square opening in the middle of roughly 1??1 cm2 dimensions. The size for this mask was chosen to match the typical
size of the MWCNT’s used for these tests. This leads us to the third curve in Fig. 6 labeled “MWCNT on Ti(t=0)”. These are data corresponding to the ratio calculated by dividing the reflected light from the sample by that of the 100% reference sample. We find the average value is around 64%, which is about 2% higher than that of the black calibrator sample.
The final step in order to obtain properly normalized THR data from the ratios shown in Fig. 6 was done by
means of the formula:
![]()
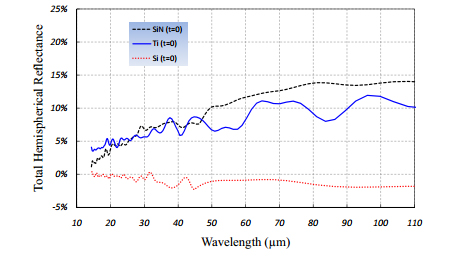
The results of applying the calculations of Eq. 9 to the data in Fig. 6, along with results of MWCNT grown on Si and SiN substrates are shown in Fig. 8. The THR values for all the samples start off in agreement with the λ 20μm data shown in Fig. 5.
However, the data for the MWCNT grown on SiN and Ti samples show a positive (almost linear) increase towards longer wavelengths, reaching values close to 14% and 10% respectively at the long-end of the wavelength range shown.
These results suggest that the these coatings are becoming less effective in absorbing electromagnetic radiation at longer wavelengths. This is in contrast with the results obtained with the MWCNT sample grown on Si substrate where the THR values remain constant near the zero mark in Fig. 8.
In other words, we found nearly identical response from the either the MWCNT sample grown on Si or the black calibrator standard. The slightly negative results shown in Fig. 8 for the Si sample are well within the margin of error and they result from directly applying Eq. 9 to the ratios taken with the MWCNT sample and the black calibrator.
The fact that a MWCNT sample made on Si substrate is a better performer in terms of low THR at far-infrared wavelengths when compared to other substrates should not be too surprising. This is because Si is the most suitable substrate on which to grow longer and aligned MWCNT when compared
to either the SiN or Ti substrates as discussed in Sec. 3.
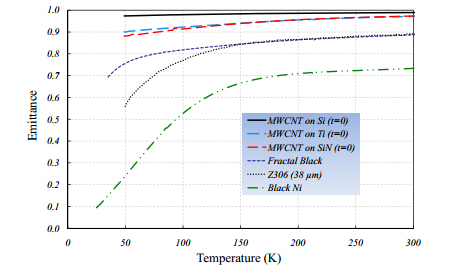
4.3 Emittance Calculations
We now turn our attention to calculating emittance versus temperature using the equations presented in Sec. 2.2. We performed these calculations for the three samples shown in Fig. 8. We also compare these results with other more traditional black coatings. The results are shown in Fig. 9.
The integral in Eq. 4 implies that the THR data would be needed over an infinite wavelength range; but in practice, it is sufficient that the upper wavelength limit for the THR data contains 95% or more of the Planck’s radiator energy. Another important consideration is that the measured THR does not have a strong dependence on temperature.
We have validated this assumption in the present case since we found the THR data at ambient (300 K) temperature merge smoothly without any discontinuity in the range they overlap with the far-infrared data taken at 7 K on the same sample.
The results of this analysis yields an integrated emittance that is the highest for the MWCNT grown on Si (t=0) substrate. The average value of ε(T ) stays around 97-98% over the 50 to 300 K temperature range shown in Fig. 9.
The MWCNT coatings grown on Ti and SiN display the second and third best emittance values as a function of temperature (average values over 95% for both samples). These averages compare favorably with the current state-of-the-art that are typically measured on Fractal black and other more commonly used black paints such as Z306.
CONCLUSIONS
In conclusion, we have performed total hemispherical reflectance (THR) measurements on MWCNT samples grown on various substrates in the visible mid- and far-infrared wavelengths.
These results show that samples prepared on Si substrate display the lowest THR value when compared to samples grown on other types of substrates across a very broad spectral range (0.2 to 110 μm). Furthermore, samples grown on Ti or SiN offer comparable performance but only up to 20 μm. The THR data for these are higher at wavelengths as high as
110 μm. Explanation for the superior performance of the Si-based sample could be due to two possibilities. First of all, if these results are explained in terms of a partially transmissive Si substrate at infrared wavelengths, the higher absorption could be due to a double-pass of the light through the nanotubes coatings.
This is due to the fact that the sample was mounted on an opaque Al disk that would have reflected back any light that had initially made it through the nanotubes and the substrate. Secondly, if nanotubes grown on Si made the sample completely opaque to infrared radiation, the high absorption would imply the nanotubes may be longer and better aligned in comparison to samples grown on either SiN or Ti substrates.
Although we believe this to be the most likely explanation, further studies would be required to finally settle this issue. But regardless of the substrate on which they are grown, the THR data measured on any of the MWCNT samples lead to emittance values that are higher across a temperature range from room temperature down to about 40 K, when compared to other more traditional black coatings such as Acktar Fractal Black or Z306.
ACKNOWLEDGMENTS
This work is supported through a NASA-Goddard Space Flight Center (GSFC) Internal Research and Devel- opment (IRAD) grant (J.G. Hagopian, PI). One of us, Raymond (Robin) E. Kinzer Jr., is supported by an appointment to the NASA Postdoctoral Program at GSFC administered by the Oak Ridge Associated Univer- sities under contract with NASA.
REFERENCES
- John G. Hagopian, Stephanie A. Getty, Manuel Quijada, June Tveekrem, Ron Shiri, Patrick Roman, James Butler, Georgi Georgiev, Jeff Livas, Cleophus Hunt, Alejandro Maldonado, “Multiwalled carbon nanotubes for stray light suppression in space flight instruments,” Proc. SPIE 7761, 77610F (2010).
- Nicodemus, F. E., Richmond, J. C., Hsia, J. J., Ginsberg, I. W., and Limperis T., [Geometrical considera- tions and nomenclature for reflectance ], National Bureau of Standards Monograph 160, US Department of Commerce, Washington (1977).
- Stephen A. Rinehart, Dominic J. Benford,1 Giuseppe Cataldo, Eliahu Dwek, Ross Henry, Raymond E.Kinzer, Jr., Joseph Nuth III, Robert Silverberg, Caleb Wheeler, and Edward Wollack, “Measuring the optical properties of astrophysical dust analogues: instrumentation and methods,” Applied Optics 50, 4115–4124 (2011).
- Zu-Po Yang, Lijie Ci, James A. Bur, Shawn-Yu Lin, and Pulickel M. Ajayan, “Experimental observation of an extremely dark material made by a low-density nanotube array,” Nanoletters 8, 446–451 (2007).
- E.J. Wollack, D.J. Fixsen, A. Kogut, M. Limon, P. Mirel, J. Singal, “Radiometric waveguide calibrators,”IEEE Transactions on Instrumentation and Measurement 56, 2073–2078 (2007).
- D.J. Fixsen, E.J. Wollack, A. Kogut, M. Limon, P. Mirel, J. Singal, J., “Compact radiometric microwave calibrator,” Review of Scientific Instruments 77, 64905–1–6 (2006).

